Discrete Mathematics

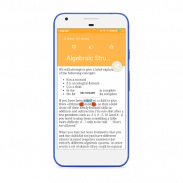
It is designed swipe based for engineering student of all streams to learn discrete mathematics. It almost cover all important topics which are covered chapter wise.

Chapter 1 Set Theory, Relation, Function, Theorem Proving Techniques

1. Set Theory

2. countable and uncountable sets

3. Venn Diagrams

4. proofs of some general identities on sets Relation in Venn Diagrams

5. types of relation

6. composition of relations

7. Equivalence relation

8. Partial ordering relation

9. one-to-one Function

10. into and onto function

11. Inverse Functions

12. Pigeonhole Principle

Chapter 2 Algebraic Structures

1. Algebraic structures

2. Abelian group

3. Subgroups

4. cyclic group

5. Homomorphism and isomorphism of Groups

6. Rings and Fields

Chapter 3 Propositional Logic

1. Proposition

2. Conditional Statements

3. Truth Tables of Compound Propositions

4. Logic and Bit Operations

5. PROPOSITIONAL EQUIVALENCES

6. Logical Equivalences

7. Constructing New Logical Equivalences

8. Predicates

9. Quantifiers

10. Infinite States and Infinite State Transitions

11. Finite state machines as language recognizers

Chapter 4 Graph Theory

1. Introduction of graphs

2. Basic Terms of Graph Theory

3. Planer graphs

4. multigraph

5. isomorphic Graph

6. paths, cycles, trails, and circuits

7. Shortest paths

8. Eulerian and Hamiltonian paths and circuits

9. Graph coloring

10. chromatic number

11. Homomorphism and isomorphism of Groups

Chapter 5 Posets, Hasse Diagram and Lattices

1. Posets, Hasse Diagram

2. ordered set

3. Hasse diagrams

4. isomorphic ordered set

5. well ordered set

6. properties of Lattices

7. bounded and complemented lattices

8. Combinatorics

9. Permutation and combination

10. Binomial Theorem

11. Introduction to Recurrence Relation and Recursive algorithms

12. Linear recurrence relations with constant coefficients

13. Homogeneous solutions

</div> <div jsname="WJz9Hc" style="display:none">มันถูกออกแบบมารูดที่ใช้สำหรับนักศึกษาวิศวกรรมลำธารทั้งหมดเรียนรู้คณิตศาสตร์ไม่ต่อเนื่อง มันเกือบจะครอบคลุมทุกประเด็นที่สำคัญที่ได้รับความคุ้มครองที่ชาญฉลาดบท

บทที่ 1 ทฤษฎี Set, ความสัมพันธ์, ฟังก์ชั่นทฤษฎีบทเทคนิคการพิสูจน์

1. ตั้งทฤษฎี

2. ชุดนับได้และนับไม่ได้

3. แผนภาพเวนน์

4. การพิสูจน์ตัวตนทั่วไปบางอย่างเกี่ยวกับชุดความสัมพันธ์ในแผนภาพเวนน์

5. ประเภทความสัมพันธ์

6. องค์ประกอบของความสัมพันธ์

7. ความสัมพันธ์ความเท่าเทียม

8. ความสัมพันธ์การสั่งซื้อบางส่วน

9. แบบหนึ่งต่อหนึ่งฟังก์ชั่น

10. เข้าและฟังก์ชั่นบน

11. ฟังก์ชั่นผกผัน

12. ซุกหลักการ

บทที่ 2 โครงสร้างพีชคณิต

1. โครงสร้างพีชคณิต

2. กลุ่มศาสนาคริสต์

3. กลุ่มย่อย

4. วงจรกลุ่ม

5. homomorphism และมอร์ฟของกลุ่ม

6. แหวนและทุ่งนา

บทที่ 3 ประพจน์ลอจิก

1. ข้อเสนอ

2. งบเงื่อนไข

3. ตารางความจริงข้อเสนอของสารประกอบ

4. การดำเนินงานของลอจิกและบิต

5. equivalences ประพจน์

6. ตรรกะ equivalences

7. สร้าง equivalences ตรรกะใหม่

8. Predicates

9. บ่งปริมาณ

10. ไม่มีที่สิ้นสุดไม่มีที่สิ้นสุดสหรัฐอเมริกาและรัฐเปลี่ยน

11. เครื่องรัฐ จำกัด เป็น recognizers ภาษา

บทที่ 4 ทฤษฎีกราฟ

1. บทนำของกราฟ

2. ข้อตกลงพื้นฐานของทฤษฎีกราฟ

3. กราฟกบ

4. multigraph

5. isomorphic กราฟ

6. เส้นทางรอบเส้นทางและวงจร

7. เส้นทางที่สั้นที่สุด

8. Eulerian และเส้นทางมิลและวงจร

9. กราฟสี

10. สีจำนวน

11. homomorphism และมอร์ฟของกลุ่ม

บทที่ 5 posets, Hasse แผนภาพและประดับประดาด้วย

1. posets, Hasse แผนภาพ

2. สั่งตั้ง

3. แผนภาพ Hasse

4. isomorphic สั่งตั้ง

5. ดีสั่งตั้ง

6. คุณสมบัติของประดับประดา

7. โปรยกระโดดและครบครัน

8. Combinatorics

9. เรียงสับเปลี่ยนและการรวมกัน

10. ทฤษฎีบททวินาม

11. รู้เบื้องต้นเกี่ยวกับการเกิดขึ้นอีกซ้ำความสัมพันธ์และขั้นตอนวิธี

12. ความสัมพันธ์เชิงเส้นที่มีสัมประสิทธิ์การเกิดขึ้นอีกอย่างต่อเนื่อง

13. การแก้ปัญหาที่เป็นเนื้อเดียวกัน</div> <div class="show-more-end">